Overview:

Many engineers affect “Probabilistic life prediction” by replacing constants with probability distributions and carefully modeling the physical relationships among the parameters. Unfortunately, the statistical relationships among the “constants” are often ignored altogether. Few recognize that while this simple substitution of distributions for constants will indeed produce a non-deterministic result, the corresponding “probabilities” are often woefully inaccurate. In fact, even the “trend” can be wrong, so these results can’t even be used for sensitivity studies.

There’s a lot more to it than that

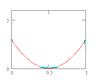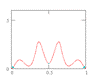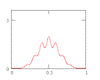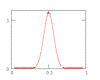
There is more to Monte Carlo simulation than replacing constants with probability densities. This is vividly illustrated by comparing the observed distribution of lives of 68 nominally identical crack growth specimens with Monte Carlo (MC) simulations of lives based on the distributions of their Paris law parameters. Several common MC sampling techniques produce wildly inaccurate results, one with a standard deviation that is 700% larger than was exhibited by the specimen lives themselves.

Because the estimates of the Paris law parameters are jointly distributed as bivariate normal, a MC simulation using this joint de

nsity reproduces the 68 specimen mean and standard deviation to within a few percent. The lessons here apply to any regression model, not just to these data, nor only to crack growth behavior.

continued …